Parser Combinators

For the task of implementing a parser, we've seen two options so far:

Hand-written recursive-descent parsers
Compiler-compilers

We now briefly discuss a third, intermediate one: parser combinators.

Parsers are Functions

From a conceptual point of view, a parser is just a function: it takes as an input a stream of characters or tokens, and either successfully consumes a part of the input and returns “success”, or fails to read a proper sequence and fails. Parser combinators are operators that combine several such parsers to build a new one.

From two parsers $A$ and $B$ , the two basic operations are:

sequencing: construct the parser $A \sim B$ that recognizes the language $L(A) \cdot L(B)$
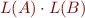
alternation: construct the parser 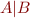$A | B$ that recognizes the language $L(A) \cup L(B)$

In a programming language that supports lazy evaluation, it is possible to implement these two operations in a very natural way. Additionally, when the language supports a form of operator overloading, it is possible to construct parsers in a way that looks very close to context-free grammars; we'll see how we can for instance write a parser for arithmetic expressions that looks like this:

    def ID : Parser = "x" | "y" | "z"
 
    def expr : Parser = factor ~ ((
      "+" ~ factor
    | "-" ~ factor
    ) | epsilon)
 
    def factor : Parser = term ~ ((
      "*" ~ term
    | "/" ~ term
    ) | epsilon)
 
    def term : Parser = (
      "(" ~ expr ~ ")"
    | ID
    | NUM
    )

Implementing Parser Combinators in Scala

See the code here. Observe how simple the definitions of Sequence and Alternative are. Some remarks on this code:

Sequence and Alternative take lazy arguments. Why?
There are no semantic actions (the parser just recognizes a string).
White spaces are not handled specially, ie. “space” is a character like any other.
Where does backtracking occur?

The second point can be improved by adding a return value as part of the Success class (ie. on success, parsers return the remaining stream and a value).

Note on the ~ combinator

The implementation of ~ as shown in the source file above does not always produce a parser that recognizes 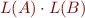$L(A) \cdot L(B)$ . As an example, consider a parser $A$ that recognizes any number of repetitions of “a”, and a parser $B$ that recognizes the words starting with one “a” and immediately followed by any number of “b”. 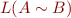The sequence “a” “a” “a” “b” will not be recognized as belonging to $L(A \sim B)$ by the combined parser, because the parser $A$ in the combination will consume all “a” and $B$ will then fail.

Scala Standard Library Implementation

The standard library includes an implementation of parser combinators (in the package scala.util.parsing.combinator). There are more combinators, for instance the * and ? operators, as well as full support for semantic actions (see the ^^ combinator).