Problem 0
Review the Exercises 06 and solve the following exercises:
Answer the remaining questions from the Problem 3 c) that were not answered during the exercise session, namely: Is 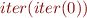 a fixpoint of
a fixpoint of 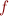 ? Is
? Is 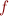 an
an  -continuous function?
-continuous function?
Problem 1
Define a monotonic function 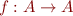 such that, for every natural number
such that, for every natural number  , the value
, the value 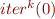 is not a fixpoint of
is not a fixpoint of 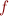 .
.