LARA

An example of a fixed point iteration not converging to a fixed point in one countable sequence

\begin{equation*} \begin{array}{l} f : [0,2] \to [0,2] \\begin{equation*}1ex] f(x) = \left\{\begin{array}{rl} \displaystyle\frac{1+x}{2}, & x < 1 \\begin{equation*}2ex] \displaystyle \frac{3+2x}{4}, & x \geq 1 \end{array}\right. \end{array} \end{equation*}

(draw figure)

Start from e.g. x=1/2. Obtain a series converging to 1, but 1 is not a fixed point because 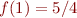$f(1)=5/4$.

This is because 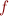$f$ is not continuous. If it was continous (at least, continuous from the left), we would have the desired property.

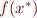Whenever we converge to some $x^*$, we take $f(x^*)$ and continue iterating and taking limits (the “number of times” to iterate, even if infinite, depends on the size of the lattice, which can be formalized ordinal numbers).